half life formula chemistry

Where t12 is the half-life of a certain reaction unit - seconds R0 is the initial reactant concentration unit - molL-1. Other isotopes have shorter half-lives.

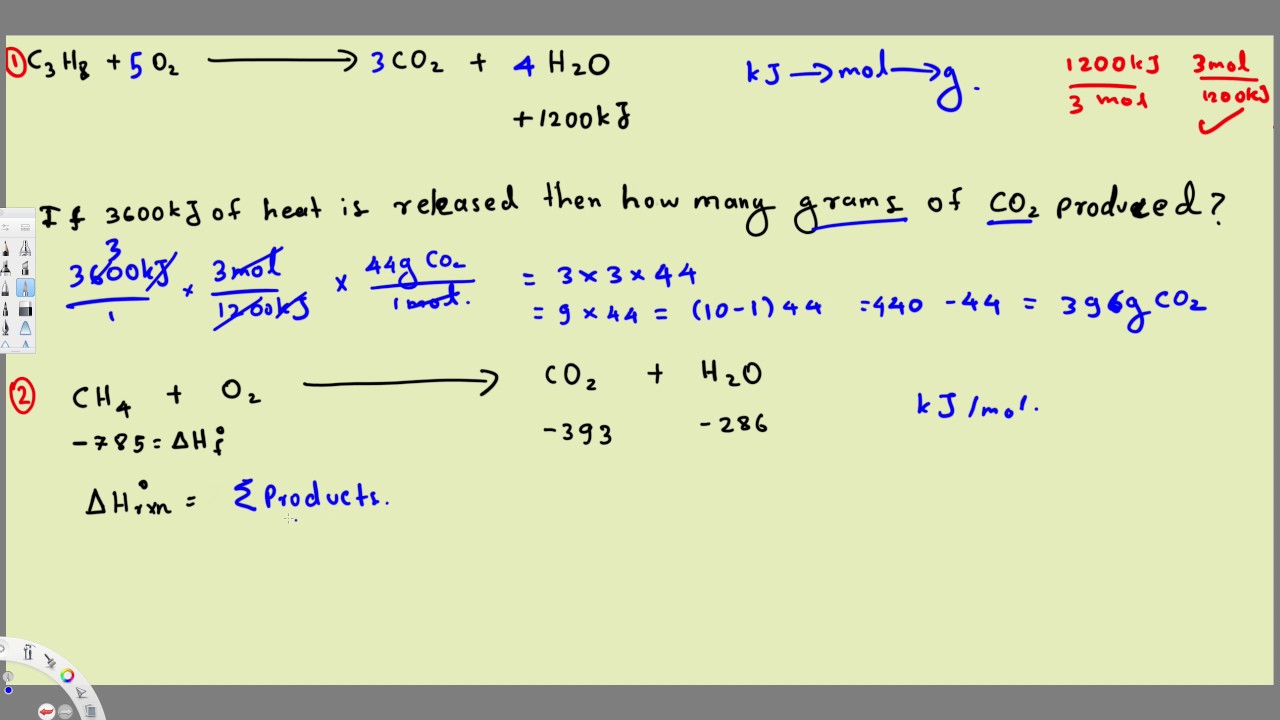
Thermochemistry Equations Formulas Practice Problems Example 2 Equations Practice Chemistry

The half-life of a reaction is the time required for the reactant concentration to decrease to one-half its initial value.

. As always lets begin with the fundamental expression Nn H1ê2Ln N0. One can describe exponential decay by any of the three formulas. The half-life of a sense is when it takes half of its initial Concentration to be used.

Determining a Half Life. An ingenious application of half-life studies established a new science of determining ages of materials by half-life calculations. And for the second-order reaction the formula for the half-life of the reaction is given by 1k R 0.

The general equation with half life. Solution t 1 2 13. Another equation you might.

T 1 2 Half life of the substance. Graphical relations and half lives. This means our y-axis values will be as follows.

Now lets think about this. In which N 0 is the number of atoms you start with and N t the number of atoms left after a certain time t for a nuclide with a half life of T. For a zero order reaction the formula is t½ Ao 2k.

The half-life of a first-order reaction does not depend upon the concentration of the reactant. N t N 0 05 t T. If an archaeologist found a fossil sample that contained 25 carbon-14 in comparison to a living sample the time of the fossil samples death could be determined by rearranging equation 1 since N t N 0 and t 12 are known.

What is its half-life. N t N 0 e -tτ N t N 0 e -λt τ is the mean lifetime - the average amount of time a nucleus remains intact. λ 0.

N t mass of radioactive material at time interval t N 0 mass of the original amount of radioactive material. The measurement of this quantity may take place in grams moles number of atoms etc. The half-life equations for a zeroth first and second order reaction can be derived from the corresponding integrated rate laws using the relationship given above.

If a sample initially contains 500 g of fluorine-20 how much remains after 600 s. Your half-life of a first. Get access to.

T time interval t 12 for the half-life. So we have the negative of that so we get a positive value here for our half life. 2λ 0693 λ.

The end product of the decay of U. So here is your half-life for a first order reaction. T 12 0693 λ.

A specific isotope might have a total count of 30000 cpm. T 12 0693k. Although similar to Example 3 the amount of time is not an exact multiple of a half-life.

It is also possible to determine the remaining quantity of a substance using a few other parameters. We know that at the half-life time eqt_12 eq the concentration of the reactant will be half as much as the initial concentration. And so your half-life is constant.

Therefore we can set eqA eq equal to eqA_02. We can also use the relation A t 1 2 n A o where n is the number of half-lives A t A o 2 n. For the first-order reaction the half-life is defined as t12 0693k.

2λ 2 0693. 5 log 2 log 325. The formula for half-life in chemistry depends on the order of the reaction.

Given that for a First Order reaction the half-life is twice the value of the rate constant find the value of the rate constant of the reaction. T 12 is the half-life τ is the mean lifetime λ is the decay constant. T ½ 1 k A o Top.

Calculate the half-life of the radioactive source. So the half-life of that isotope is one hour. Substituting into the equation.

The half-life of a reaction t 1 2 is the time required for an initial reactant concentration A 0 to decrease by one-half. Where N0 refers to the initial quantity of the substance that will decay. In this case we know that in 20.

The half-life is a valuable concept in chemistry. Let the rate constant be λ. 2 270 days.

Some isotopes have long half-lives the half-life of U-234 is 245000 years. For a first order reaction t½ 0693 k and for a second order reaction t½ 1 k Ao. λ 2 03465.

789 h o u r s. For a zero order reaction A products rate k. T ½ 0693 k For a second order reaction 2A products or A B products when A B rate kA 2.

We use the equation A t 1 2 t t 1 2 A o where A t is the activity in time t A o is the original activity 500 1 2 10 t 1 2 6000 t 1 2 10 log 2 log 12 2. Calculate the half-life of Gold-198 given that 3257 mg of this radioactive isotope decayed to 102 mg in 135 days. For each half-life that occurs the amount of In-115m decreases by half of the previous point.

In this case the half-life of a chemical is the number of years needed to metabolize 50 of it. N t N0. Solving for n we get-n logH2LlogH010LlogH10μ10-1L-1 n têthalf 1êlogH2L1ê03020 minêthalf.

We can determine the amount of a radioactive isotope remaining after a given number half-lives by using the following expression. This term is often used in radioactivity where it is used to estimate the age of rocks and other materials. Then write the half-life equation as.

N t N0. T ½ A o 2k For a first order reaction A products rate kA. In which N0 is the number of atoms you start with.

N t N0. It is a constant and related to the rate constant for the reaction. T is the half-life.

The half-life of fluorine-20 is 110 s. This expression works best when the number of half-lives is a whole number. Min H1ê2Ln Nn ÅÅÅÅÅÅÅÅÅÅ N0 010 N0 ÅÅÅÅÅÅÅÅÅÅÅÅÅÅÅÅÅÅÅÅÅÅÅÅ N0 010.

This means that the fossil is 11460 years old. Therefore A t 1 2 A 0 at t 1 2. Where n is the number of half-lives.

If k is a constant obviously 693 is a constant. The half-life of U -238 is 45 109 years. 25 125 625 3125 15625.

The half-life of fluorine-20 is 110 s. Half-life can also be expressed interms of the number of half-lives n and total time t as in the equation below. In one hour the count could be 15000 cpm half the original count.

Here we identify the initial amount as 500 g t 600 s and t 12 110 s. For geological dating the decay of U -238 can be used. Half-life or t½ is the time that elapses before the concentration of a reactant is reduced to half its initial value.

K decay constant. Then half-life t 12 2λ. Equations for Half Lives.

You can replace the N with the activity Becquerel or a dose rate of a substance as long as you use the same units for N t and N 0. In nuclear chemistry radioactive half life is defined for a simple radioactive decay process as the time required for the activity to decrease to half its value by that process. So our half-life is equal to let me rewrite this here so our half-life t 12 is equal to 693 divided by k where k is our rate constant.

The general equation with half life NtN005tT.

Basic Trigonometry Formulas Math Formula Chart Trigonometry Math Formulas

Half Life Calculator Real Life Math Half Life Learning Techniques

Chapter 6 Oxidation Reduction Redox Reactions Teaching Chemistry Chemistry Study Guide

Ch 5 5 Multiple Angle And Product To Sum Formulas Ppt Download Free Math Help Trigonometry Word Problem Worksheets

Zero Order Kinetics Reactions Online College Chemistry Courses College Chemistry Online Chemistry Courses Physical Chemistry

Half Reaction Easy Science Redox Reactions 10th Grade Science Easy Science

Atomic Size Order Chemistry Math Atom

Condensing Logs Logarithmic Functions Functions Math Organic Chemistry Study

Kinetics Ppt Chemical Kinetics Chemical Equation Enzyme Kinetics

Calculation Of Half Life Of Radioactive Substances Half Life Physics Formulas Life

Mass Defect And Binding Energy Binding Energy Chemistry Energy

Radioactive Decay And Half Life Chemistry Lessons Chemistry Classroom Chemistry Lesson Plans

Chemical Kinetics And Half Life Online College Chemistry Courses Chemical Kinetics Study Chemistry Half Life

Zero Order Kinetics Chemistry Textbook Chemistry Classroom Chemistry Experiments

Half Life Formulla Half Life Life Radioactive

Half Life Practice Worksheet Answers A Worksheet Is Often A Small Note Distributed By A Tutor To Students Tha Half Life Persuasive Writing Prompts Worksheets